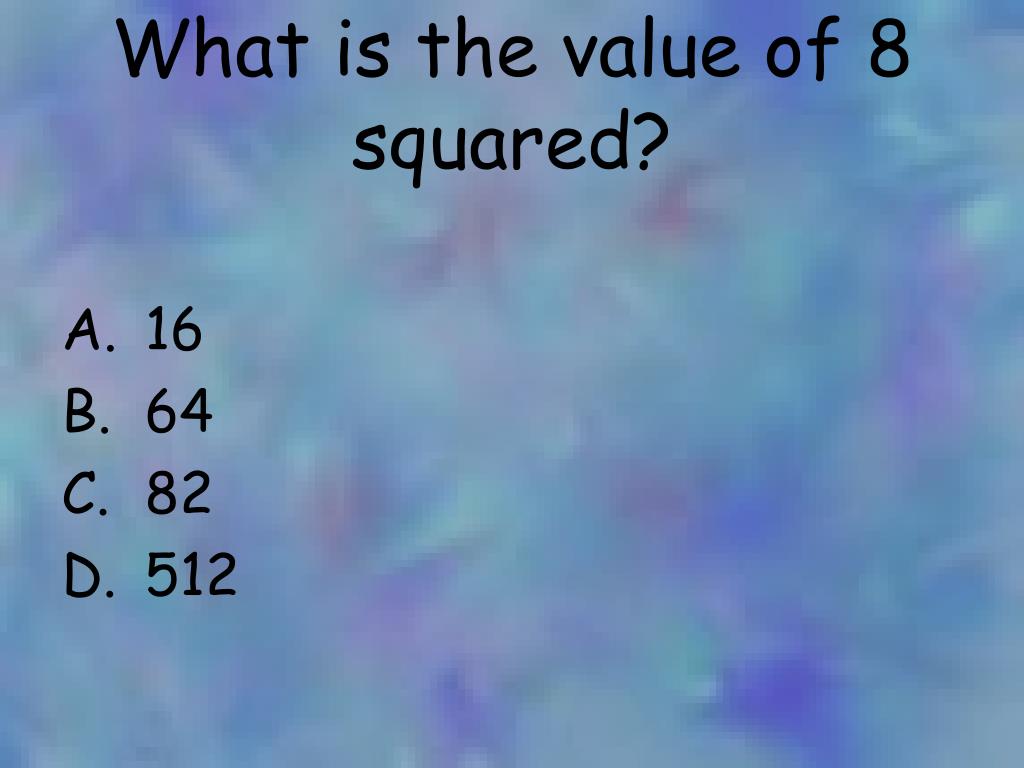What Is 8 Squared? Formula, Calculator & Examples!

Ever wondered how seemingly simple mathematical concepts unlock complex problem-solving? Understanding "8 squared" is more than just memorizing a number; it's grasping the foundation of exponents, areas, and much more.

At its core, squaring a number means multiplying it by itself. For example, to find the squared value of any number, positive or negative, you simply multiply that number by itself. This principle is universal and applies regardless of whether the number is an integer, a decimal, or even a fraction. When we talk about "8 squared," what we mean is 8 multiplied by 8. This is often written as 8², where the superscript 2 indicates the exponent. So, 8² = 8 8 = 64. This seemingly basic calculation has far-reaching implications in various fields, from geometry to computer science.

When confronted with the question, "What is 8 squared?", visualizing a square can be incredibly helpful. Imagine a square where each side measures 8 units in length. The area of this square is precisely what we're calculating when we square 8. The area of a square is found by multiplying the length of one side by the length of another side. In this case, it's 8 units 8 units, which equals 64 square units. Therefore, the answer to "what is 8 squared?" is 64. It's a fundamental concept to remember that "squared" signifies that the exponent is 2, irrespective of the base number.

• Mustsee Indian Erotic Web Series Your Ultimate Guide
• Your Guide To Flyertalk Miles Points And Travel Secrets Unveiled

The concept of squaring numbers extends beyond just simple multiplication. It's deeply rooted in geometry, especially when dealing with areas of squares and rectangles. When calculating the area of a square or rectangle, we often need to square the length of one of the sides. This is because the area of a rectangle (or square) is the product of its two dimensions. In the case of 8 squared, if we have a square with sides of length 8 units, the area of the square would be 64 square units. This application is not just theoretical; it's practical in fields like architecture, engineering, and design, where calculating areas is a common task.

Exponents, including the concept of squaring, are crucial for simplifying multiplications. They provide a concise way to represent repeated multiplication of the same number. Instead of writing 8 8, we can simply write 8². Understanding exponents is vital for more complex mathematical operations, including polynomials, exponential functions, and scientific notation. Learning what exponents are and how to use them to simplify multiplications is a gateway to more advanced mathematics.

It's also important to understand how to calculate positive, negative, and zero exponents. While 8² is straightforward, consider exponents such as 8^-2 or 8⁰. A negative exponent indicates the reciprocal of the number raised to the positive exponent. For example, 8^-2 = 1 / 8² = 1 / 64. Any non-zero number raised to the power of 0 is 1. Therefore, 8⁰ = 1. Understanding these rules is essential for working with exponents in various mathematical and scientific contexts. We can find examples of 8 squared and other powers can be found in various scientific and mathematical models.

• Justina Valentine Bio Zodiac Wild N Out More Facts
• 2025s Mustsee Web Series Whats New Amp Next In Streaming

Furthermore, it's beneficial to familiarize yourself with the squared symbol (²). This superscript 2 is commonly used to denote that a number is being raised to the power of 2. It's not just limited to mathematical notation; it's also used in various fields, including physics and engineering, to represent squared units, such as square meters (m²) or square inches (in²). A complete guide to the superscript 2 symbol would cover its various applications and how to properly use it in different contexts.

The term "8 squared" can be pronounced in different ways. It is usually pronounced "eight squared," "eight to the power of two," or "eight to the second power." Understanding these different pronunciations is useful for effective communication in mathematical and scientific discussions. Additionally, when dealing with negative numbers, such as -8, squaring the number means multiplying -8 by -8. The term is usually pronounced "negative eight times negative eight" or "negative eight squared." Since a negative number multiplied by a negative number results in a positive number, (-8)² = 64.

Online square calculators can be valuable tools for quickly finding the square of any real number. These calculators eliminate the need for manual calculation and are particularly useful when dealing with large or complex numbers. To use a square calculator, simply enter the value you want to be squared, whether it's an integer or a decimal, and the calculator will instantly display the squared value. These calculators are widely available online and can be a convenient resource for both students and professionals. This square calculator can easily calculate the squared value of given number. Enter the value to be squared whether an integer or a decimal, and the calculator will calculate the squared value.

If you're using software or applications that involve mathematical calculations, it's essential to ensure that the software is up-to-date. Try installing any available software updates to ensure accurate calculations and compatibility with the latest mathematical standards. Outdated software may contain bugs or errors that can affect the accuracy of your calculations.

Squared text symbols can be easily copied and pasted for various purposes. These symbols are particularly useful when you need to represent squared units or exponents in digital documents or online communications. There are various websites and tools that provide ² and other mathematical symbols that you can easily copy and paste. \ud83d\udccb squared text symbols to copy and paste. To copy the specific symbol to your clipboard, just click on it!

Need to find the square of a number? It's a straightforward process. Finding a number's square is as simple as multiplying it by itself. This principle applies to all types of numbers, including integers, decimals, and fractions.

To square fractions, you need to find the squares of both the numerator and the denominator separately. For example, if you want to square the fraction 3/4, you would square both 3 and 4. 3 squared is 9, and 4 squared is 16. Therefore, (3/4)² = 9/16. Then, reduce or simplify the result if possible to get your final answer. Squaring fractions is a common operation in various mathematical contexts, including algebra and calculus.

The concept of squaring numbers is also relevant in iterative calculations. In iterative processes, you may start with an initial value and repeatedly apply a function to get closer to a desired result. For example, in a certain calculation, our first attempt got us from 4 to 3.25; going again (b to e) gets us: 3.163 going again (b to e) gets us: 3.1623 and so, after 3 times around the answer is 3.1623, which is pretty good, because: This process can involve squaring numbers at each step to refine the approximation.

Understanding the concept of "8 squared" is essential for solving real-world problems. For example, consider this question: What is the area of a square that is 8 meters on a side? This is a direct application of squaring a number to find the area of a square. Since the area of a square is the length of one side squared, the area of a square with sides of 8 meters is 8² = 64 square meters. How big is a 8 meter square?

In summary, understanding "8 squared" involves several key concepts: It means multiplying 8 by itself, resulting in 64. It represents the area of a square with sides of length 8. It is an example of an exponent, where the exponent is 2. It is a perfect square, which is the result of squaring an integer. In 8 2 the 2 says to use 8 twice in a multiplication, so 8 2 = 8 8 = 64. 8 2 could be called 8 to the power 2 or 8 to the second power, or simply 8 squared The exponent of a number says how many times to use the number in a multiplication. 8 2 = 8 8 = 64 in words: 8 2 can be called 8 to the second power, 8 to the power 2. It's a foundational concept in mathematics with applications in various fields.

The concept is visually represented by filling an 8 by 8 square with colorful shapes. This helps illustrate that 8 squared represents the total number of units within the square. Fill the 8 by 8 square with colorful shapes. Complete any row or column of eight to make room for more.

In mathematics, a quadratic equation is an equation that can be rearranged in standard form as ax² + bx + c = 0, where the variable x represents an unknown number, and a, b, and c represent known numbers, where a 0. In mathematics, a quadratic equation (from latin quadratus 'square') is an equation that can be rearranged in standard form as displaystyleax+bx+c=0, where the variable x represents an unknown number, and a, b, and c represent known numbers, where a 0.

When performing mathematical calculations, it's crucial to follow the order of operations. This is often remembered using acronyms like PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction), BEDMAS (Brackets, Exponents, Division and Multiplication, Addition and Subtraction), BODMAS, GEMDAS, and MDAS. These acronyms provide a guide to the sequence in which operations should be performed to ensure accurate results. Solve math problems using order of operations like pemdas, bedmas, bodmas, gemdas and mdas. (pemdas caution) this calculator solves math equations that add, subtract, multiply and divide positive and negative numbers and exponential numbers.

In geometry, a square is a two-dimensional figure with four equal sides and four right angles. A square is a two dimensional figure which has its two edges of equal length. The area of a square is given by the product of its two dimensions. For example, if a square has its edge length equal to a centimeters the area of the square is given by the product a a which is equal to a 2.

For example, when calculating the area of a square or rectangle, we often need to square the length of one of the sides. In the case of 8 squared, if we have a square with sides of length 8 units, the area of the square would be 64 square units.